Relative Sizes of the Neutron, Proton and Electron

Onar Åm, who prompted me to embark on my journey into physics some seven years ago, has repeatedly criticized me for a lack of formulas and calculations in my work. I'm of the opinion that a model should be well hammered out before there's much need for detailed analysis, so I have largely ignored him.

However, I did make an analysis of my model to see if it conforms to Coulomb's law, and I had multiple situations in which my model yielded real world predictions. I was for instance delighted to learn about the Faraday Effect, which confirmed my conclusion that magnetism is a form of polarized light.

But there are other things that I haven't looked much into, which I probably should at this point, with my model now pinned down. My work is to a great extent based on Morton Spears’ particle quanta, and his calculations related to the relative sizes of the proton and neutron, to which I've added calculations for the size of the electron, positron and neutrino.

However, I never checked the validity of Morton Spears' numbers. I found this detail irrelevant for my overall thesis. My thinking was that my logic would apply to any set of numbers. The only difference in outcome would be the specific sizes of particles. But now that my model is pinned down, the time has come to look closer at Morton Spears' numbers to get the exact relative size of the four stable particles derived from my model.

In doing this, it should be noted that the proton has recently been measured to be smaller than what was thought in Morton Spears' time. The man must therefore be excused for any deviation between currently accepted numbers and numbers presented by him in his second book on gravity.

Searching the web for fresh numbers we find that the exact relative mass of the neutron, proton and electron are:

• Neutron = 1
• Proton = 0.99862349
• Electron = 0.00054386734

If we add the electron to the proton, we get 0.99916735734. That's less than a neutron by 0.00083264266. We're missing mass, equivalent to more than an electron. This is binding energy converted to kinetic energy when a free neutron decays into a proton, electron and anti-neutrino. We cannot therefore expect our relative numbers to be precise down to the last digit.

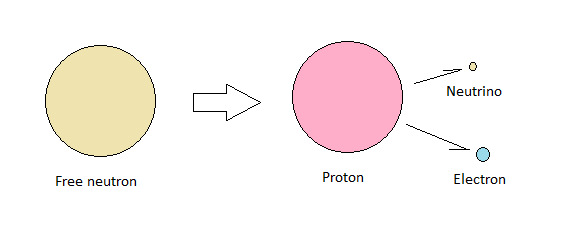Free neutron decay

With this in mind, we can make our calculations:

• We get that the neutron is 1838.68 times more massive than an electron.
• We get that the proton is 1836.15 times more massive than an electron.
• We get that the difference between a neutron and proton is 2.54 units.

From this, we see that the electron comes out a little lighter than we would have liked, and the proton comes out a little on the heavy side. But the difference is negligible, especially in light of the uncertainties related to the exact mass of these things. We can therefore say that the electron consists of 3 units, the neutron consists of 1839 units and the proton consists of 1836 units.

This compares to Morton Spears' numbers as follows:

• MS' neutron = 2180 units; modern neutron = 1839 units; a difference of 341 units.
• MS' proton = 2177 units; modern proton = 1836 units; a difference of 341 units.
• MS' electron = 3 units; modern electron = 3 units; a difference of 0 units.

When we compare these particles in terms of elementary building blocks of 3 (the size of an electron), we get that:

• MS' neutron = 726 blocks and a rest of 2 units; modern neutron = 613 blocks and 0 rest.
• MS' proton = 725 blocks and a rest of 2 units; modern proton = 612 blocks and 0 rest.

Note that the size of an electron in terms of particle quanta does not translate directly into size in terms of mass. Current numbers suggest that a neutron is 1839 times more massive than an electron. Yet it's only made up of 613 times as many blocks. The reason for this discrepancy can be ascribed to binding energy which we have already seen to be a substantial part of the neutron's mass.

The discrepancy between Morton Spears' numbers and modern numbers are due to the fact that the proton is less massive than what was accepted as fact in Morton Spears' time.

However, there's a more serious problem at hand. The proton should consist of an odd number of building blocks, so as to account for its net charge of 1. We cannot have a proton made up of an even number of blocks. Hence, its size must be either more or less than 612 blocks. The same goes for the neutron which must consist of an even number of blocks in order to produce a net charge of 0.